Measurements: units and errors are as important as the number!
By A. Zabludoff
| Units Used in Astronomy | |
| Name | Scale: typical size of … |
| 1 fermi (fm) = 10-15 m | atomic nuclei |
| 1 nanometer (nm) = 10-9 m | atoms |
| 1 meter (m) | humans |
| 1 kilometer (km)= 103 m | cities |
| 1 Astronomical Unit (AU)=1.496 x 1011 m | sun-planet distances |
| 1 light year (ly) = 9.461 x 1015 m | distances between stars |
Astronomy spans 30 orders of magnitude! You need to place 1,000,000,000,000,000,000,000,000,000,000 protons side by side to reach from the Sun to Proxima Centauri, the nearest star!
- qualitative (“It seems to have gotten cooler!”) vs. quantitative (“It’s 20 degrees F colder today than yesterday.”)
- number (amount) and units (type of quantity being counted) — “It was 80 degrees yesterday” is meaningless unless you know whether it was 80 degrees Celsius or Fahrenheit or Kelvin
- scientific (or exponential) notation — nearest star is 40,000,000,000,000 km or 4 x 1013 km away
 Fundamental properties: mass (kg), length (m), time (s)
Fundamental properties: mass (kg), length (m), time (s)
- speed, force, temperature, energy, and other properties can be derived from mass, length, and time
- example: speed = length/time [m/s]
- example: temperature = mass(length/time)2 [kg(m/s)2]
Great range of properties in astronomy
- temperature can range from 3 degrees Kelvin (empty space) to 109 degrees Kelvin (supernova shock!)
- size can range from 10-6 m (microscopic dust grains) to 1023 km (distance light has traveled in age of Universe!)
Precision vs. Accuracy
| Precision | Accuracy |
| reproducibility | correctness |
| check by repeating measurements | check by using a different method |
| poor precision results from poor technique | poor accuracy results from procedural or equipment flaws |
- precision = how many decimal places (significant figures) can you determine through your measurement,how well you think that you’ve done in making the measurement
- accuracy = amount by which measurement deviates from true value, how well you’ve actually done in making the measurement
- consider a ruler marked in cm vs. one marked in mm
- you can get a more precise measurement from the mm ruler
- but, if the ruler is marked incorrectly (say, the spacings between marks vary), your measurement cannot be accurate
Observations and Errors
observational error is uncertainty in a measurement
- example: inserting a card halfway down the deck
- 10 trials by guessing middle of deck: 24, 33, 28, 27, 23, 27, 24, 32, 26, 31, large range in values —> big error due to rough measurement
- 10 trials by measuring height of deck with mm ruler: 25, 28, 24, 27, 27, 26, 28, 26, 25, 27, smaller range in values —> less error due to careful measurement
- can give the result of several measurements of same thing (like midpoint of deck) as average and standard error
- standard error is the spread in the measurements
- not the same as a bad measurement
- represents limit to our uncertainty as result of measurement
- becomes smaller when average becomes more reliable either due to more precise measurements or increasing the number of measurements (smaller when deck measured with mm ruler, smaller when number of deck measurements increases)
random errors (measurements evenly distributed about true value) vs. systematic errors (all measurements off in one direction, bad measurement)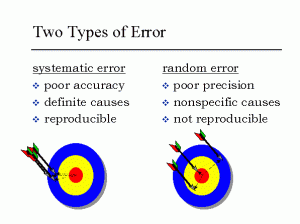
Estimation -approximation is very useful
- scientists often make estimates to get a sense of the problem without extensive calculations or book research
- example: How long before Pioneer 11 reaches the nearest stars?
- Pioneer’s speed = 100,000 or 105 km/hr
- distance to alpha Centauri = 4 x 1013 km
- so, number of hours = 4 x 1013 km / 105 km/hr =
4 x 108 hr = 4 x 108 hr (1 day/24 hr) (1 yr/365 day) =
5 x 104 yr = 50,000 years!
Logic and Mathematics
- Deduction

- premises lead to a conclusion for a particular case
- advantage: with accurate premises, conclusion is right every time
- disadvantage: conclusion contains no more information than premises
- Induction

- specific observation used to infer a general, widely applicable conclusion
- advantage: get a lot of info from only one observation (leads to good hypotheses)
- disadvantage: conclusion not guaranteed